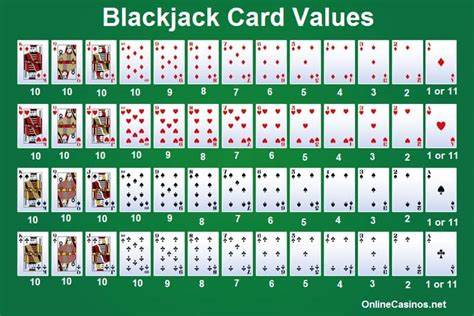

The Probability of Getting a Blackjack
Naturals are the most potent combination you can get. With a natural in your disposal, not only it ensures your win in most of the cases, but you’ll get 1.5 times your original bet. This is why understanding the probability of getting a blackjack is essential.
If you know the number of card decks in play, you can effortlessly determine the possibility of receiving a natural. For this purpose, you have to multiply the probability of getting an ace with the probability of pulling a ten-valued card, i.e., 10, J, Q, K. You also have to double up the result to get the actual probability. There are two probable combinations of the card in a Blackjack hand, for example, A-K and K-A.
4/52 is the probability of getting an ace while the possibility of pulling a ten-valued card from the rest of the deck in 16/51. Therefore, the probability of getting a natural in the first hand itself is

- 2*P(ace)*P(10-valued cards)
- 2*4/52*16/51 = 0.0482 or 4.82%
When playing Blackjack, understanding the probabilities can help improve your strategy. Here are some key probabilities to keep in mind:
Probability of Busting
| Player Hand | Probability of Busting |
| 12 | 31% |
| 13 | 39% |
| 14 | 56% |
| 15 | 58% |
| 16 | 62% |
Conclusion
Probability of Blackjack
| Probability of Being Dealt a Blackjack | 4.8% approximately 1/20 |
Playing for the Jackpot / Side Bet
| Calculations | Results |
|---|---|
| (player bj with king) / 4 | 1/80 |
| (player bj same family) / 4 | 1/320 |
| (casino bj) / 20 | 1 / 6400 |
| (casino bj with king) / 4 In addition it’s not clearly clear whether the sequence of the cards does not have to be taken into account ! | 1 / 25’600 winning the jackpot |
| bet x 5.- | 128’000.- “break even” |
